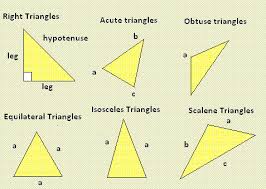
The scalene triangle is a special type of triangle that has no equal sides. It will always have three different lengths for its sides, and those lengths are unequal. In this blog post, we will discuss what a scalene triangle is as well as an acute angle.
Scalene triangle: This kind of a triangle is a triangle in which all three sides are of different lengths, and not both angles are the same. The longest side is opposite the largest angle, the shortest side is opposite the smallest angle, and the middle side is opposite the medium-sized (or “average”) angle.
Acute angle: An acute angle is an internal (between two lines) angle that measures less than 90°. For example, an acute-angled triangle has each of its angles measuring more than 30° but less than 90°. Because there are many special triangles with angles up to 180°, where some measure 45°, 30°, 42°, etc. Every acute-angled triangle can be divided up into smaller triangles by extending one line through the opposite vertex and using the line as a segment of a hypotenuse (longest side).
A right triangle with an acute angle is called an acute-angled triangle. The longest side of an acute-angled triangle that is not adjacent to the acute angle is on the side of the longest side of the original triangle and is called a “leg”. For example, in ∆ABC (shown on the right), we know AB = AC; it follows that BC > AB.
Properties:
- Scalene triangle is a type of triangle in which all three sides are of different lengths, and no two angles are the same.
- The middle side is opposite the medium-sized (or “average”) angle.
- An acute angle is an internal (between two lines) angle that measures less than 90°.
- An acute-angled triangle has each of its angles measuring more than 30° but less than 90°.
Because there are many special triangles with angles up to 180°, where some measure 45°, 30°, 42°, etc., every acute-angled triangle can be divided up into smaller triangles by extending one line through the opposite vertex and using the line as a segment of a hypotenuse (longest side).
A right triangle with an acute angle is called an acute-angled triangle. The longest side of an acute-angled triangle that is not adjacent to the acute angle is on the side of the longest side of the original triangle, and is called a “leg.”
1. The formula of a scalene triangle:
a^2 = b^2 + c^2 – 2bc cosA
2. The formula of acute angle:
a/sinA * h = b/sinB * h = c/sinC * h
Where A, B, and C are the angles of the triangle. their perpendicular to sides a,b,c that meet at A,b,c are the lengths of the sides opposite to angles A, B, and C.
Example of an acute triangle (a right triangle with an acute angle is called an acute-angled triangle.):
Trees stand at angles to one another in forests around planet Earth – this means that every forest has its unique geometry! The trees must compete for sunlight, and can tilt or curve their branches to maximize the amount of sunlight they receive.
By knowing that AC = AB = BC, we can conclude that two intersecting lines form ABC. We know BC > AB and therefore AC > AB. Since the angles are not 90°, the sum of all three angles must be greater than 180°. Therefore, ∆ACB + ∆BCA + ΔCAB >180° . The shortest side of a right-angled triangle is opposite to the 90-degree angle. So in this case, it would be AC since it’s shorter than both sides in length. This means that CB is also longer than AB because they are also two sides of a right triangle. Last, we know that all the angles in any triangle are 180° minus the smallest angle. So because there are two angles greater than 90°, the smallest angle would be less than 90°. Therefore, 30° is the value of ΔCAB, meaning ∠BAC = 30°, meaning AC is also shorter than BC, making it an acute-angled triangle.
One can always learn more about angles and many more math topics on the Cuemath website. It is an online platform that provides classes on math and coding.