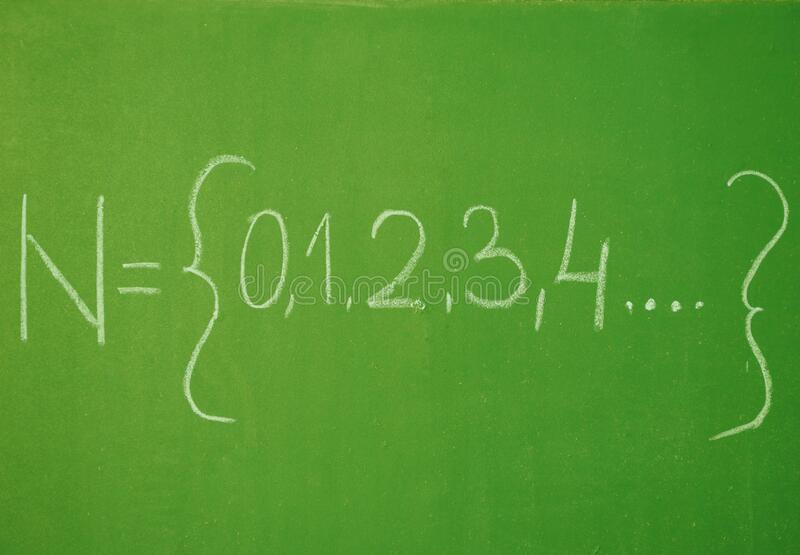The sum of n natural numbers can be written using the following number series.
What is the sum of this infinite series? Before reading any further, give yourself a moment to think. If you have not seen such a series before, and the topic of numerical series as a whole is not too close to you, then the answer to this question will be a big surprise for you.
This, at first glance, completely counterintuitive result, nevertheless, can be rigorously proved. But before talking about the proof, you need to digress and remember the basic concepts.
Let’s start with the fact that the “classical” sum of a series is the limit of partial sums of a series if it exists and is finite. Details can be found on wikipedia and related literature. If there is no finite limit, then the series is said to be divergent.
For example, the partial sum of the first k members of the number series 1 + 2 + 3 + 4 + … is written as follows
It is easy to understand that this amount grows indefinitely as k tends to infinity. Consequently, the original series is divergent and, strictly speaking, has no sum. There are, however, many ways to assign a final value to diverging series.
The row 1 + 2 + 3 + 4 +… is far from the only one of the diverging rows. Take, for example, the Grandi series
which also diverges, but it is known that Cesaro’s summation method allows you to assign a final value of 1/2 to this row. Cesaro summation consists in operating not with partial sums of a series, but with their arithmetic means. Allowing ourselves to speculate in a freestyle, we can say that the partial sums of the Grandi series oscillate between 0 and 1, depending on which term of the series is the last in the sum (+1 or -1), hence the value 1/2, as arithmetic average of two possible values of partial sums.
Another interesting example of a divergent series is the alternating series 1 – 2 + 3 – 4 + …, whose partial sums also oscillate. Abel summation allows you to assign a final value of 1/4 to a given series. Note that Abel’s method is, in a way, a development of the Cesaro summation method, so the result 1/4 is easy to comprehend from the point of view of intuition.
It is important to note here that summation methods are not tricks that mathematicians have invented to somehow cope with diverging series. If you apply Cesaro summation or Abel’s method to a converging series, then the answer given by these methods is equal to the classical sum of a converging series.
Neither Cesaro summation nor Abel’s method, however, allow working with the series 1 + 2 + 3 + 4 + …, since the arithmetic means of partial sums, as well as the arithmetic means of arithmetic means, diverge. In addition, if the values 1/2 or 1/4 can somehow be accepted and correlated with the corresponding series, then -1/12 is difficult to associate with the series 1 + 2 + 3 + 4 + …, which is an infinite sequence of positive whole numbers.
There are several ways to arrive at a -1/12 result. In this post, I will only briefly dwell on one of them, namely the zeta function regularization . Let’s introduce the zeta-function
Substituting s = -1 , we get the original number series 1 + 2 + 3 + 4 +…. Let’s do a number of simple mathematical operations on this function.
Where imageis the Dirichlet eta-function
When s = -1, the eta-function becomes already familiar to us by the series 1 – 2 + 3 – 4 + 5 – … whose “sum” is equal to 1/4. Now we can easily solve the equation.
Interestingly, this result finds its application in physics. For example, in string theory. Turning to page 22 of Joseph Polchinski’s “String Theory”:
If for some, string theory is not a convincing example due to the lack of evidence of many of the consequences of this theory, then we can also mention that similar methods appear in quantum field theory when trying to calculate the effect Casimir.
In order not to walk twice, a couple more interesting examples with the zeta function
For those who want to get more information on the topic, I note that I decided to write this note after translating the corresponding article on Wikipedia , where in the “Links” section you can find a lot of additional material, mainly in English.
Read More Articles on Evoking Minds